| |
 |
 |
 |
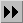 |
 |
 |
 |
 |
 |
B.2.3 Global orderings
For all these orderings, we have Loc ![]() =
= ![]()
- lp:
- lexicographical ordering:
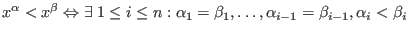 .
. - rp:
- reverse lexicographical ordering:
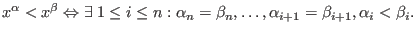
- dp:
- degree reverse lexicographical ordering:
let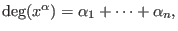 then
then
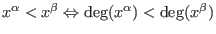 or
or
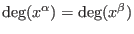 and
and
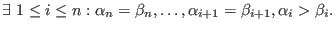
- Dp:
- degree lexicographical ordering:
let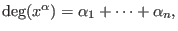 then
then
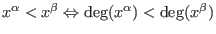 or
or
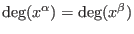 and
and
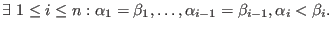
- wp:
- weighted reverse lexicographical ordering:
let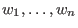 be positive integers. Then
be positive integers. Then
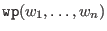 is defined as
is defined as dpbut with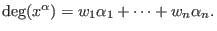
- Wp:
- weighted lexicographical ordering:
let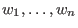 be positive integers. Then
be positive integers. Then
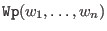 is defined as
is defined as Dpbut with