For applications to D-modules and to vector bundles over projective spaces the computation of standard bases and syzygies in two special non-commutative algebras is also implemented in SINGULAR. The advantage of SINGULAR having almost all possible monomial orderings implemented allows us to compute in the local and in the global case.
SINGULAR can compute the standard basis of modules over the (non-commutative)
algebra
![]() with the relations
with the relations
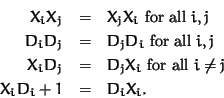
The Di may be considered as differential operators.
The only restriction we have to make to the ordering is the assumption that
L([m, m']) < L(m, m') for all monomials m, m' with
![]() .
Especially a product ordering with the property that monomials in the Di
are always greater than monomials in the Xi and which is a wellordering on
.
Especially a product ordering with the property that monomials in the Di
are always greater than monomials in the Xi and which is a wellordering on
![]() ,
is admissible. Hence, SINGULAR can compute standard
bases in (Loc
,
is admissible. Hence, SINGULAR can compute standard
bases in (Loc
![]() )
)
![]() ,
in particular in
the Weyl algebra
,
in particular in
the Weyl algebra
![]()
![]() and in the
``local Weyl algebra''
and in the
``local Weyl algebra''
![]()
![]() .
Moreover, we have implemented standard basis and syzygies of
modules over the algebra (Loc
.
Moreover, we have implemented standard basis and syzygies of
modules over the algebra (Loc
![]() )
)
![]() with the relations
with the relations
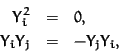
in particular over the tensor product of
![]() with the
exterior algebra.
with the
exterior algebra.