


Next: Computing the divisors
Up: Computational Aspects
Previous: Computing the places of
Computing the adjunction divisor
For any (closed) singular place we determine the multiplicity
![$ d_{[Q]}:=ord_Q ({\mathcal{A}})$](img479.gif) , alternatively, by the formula (2)
or the Dedekind formula (cf. Remarks 2.5 and 2.3). Note that to compute a local intersection number
, alternatively, by the formula (2)
or the Dedekind formula (cf. Remarks 2.5 and 2.3). Note that to compute a local intersection number 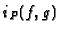 (as
appearing in both formulas), we can proceed inductively,
computing the primitive parametrization
(as
appearing in both formulas), we can proceed inductively,
computing the primitive parametrization
![$ \varphi:K[[x,y]]\to
K[[t]]$](img481.gif) of
of 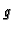 up to degree
up to degree 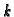 , until
, until
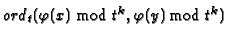 is less than
is less than
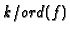 .
.
Christoph Lossen
2001-03-21